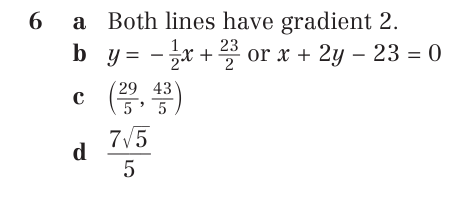Armul Mathematics & Computing
Armul Mathematics & Computing
Resources on this page:
SBD with worked solution | Video going through an SBD question | Key points | Interactive used in the video

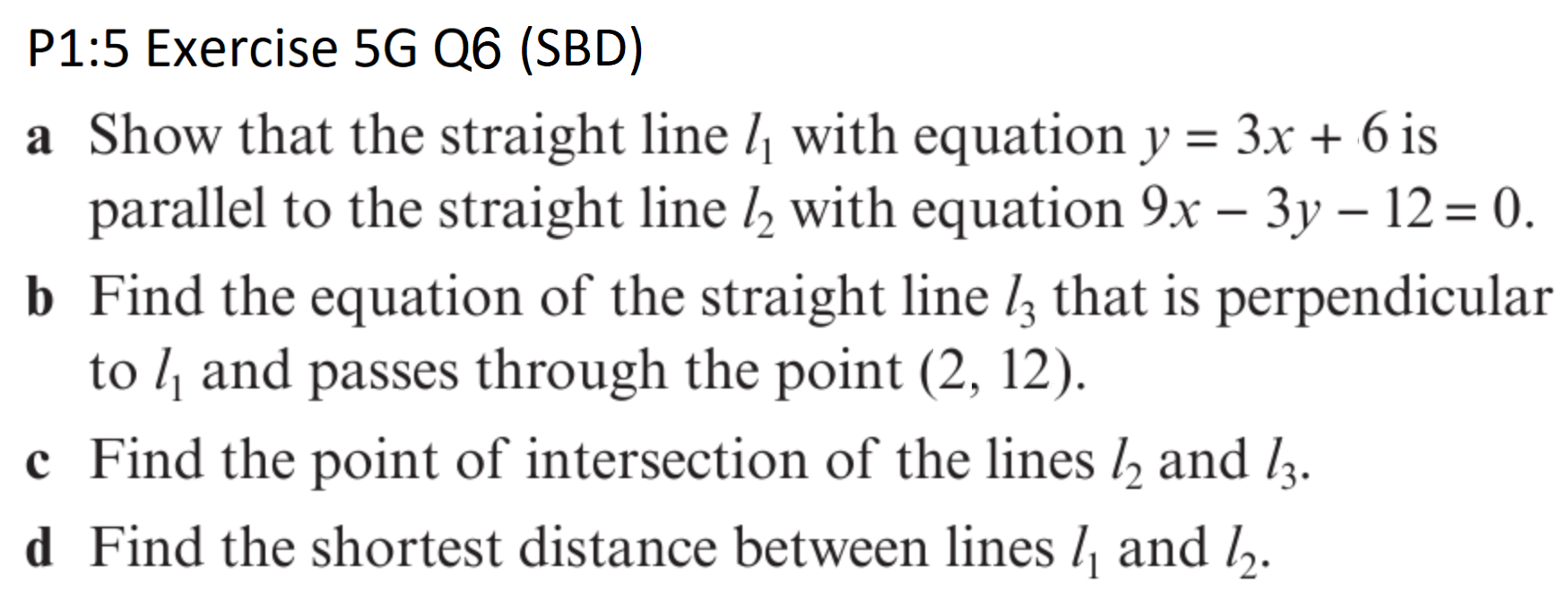
P1.5G Q6 - the answer is at the bottom of this page


You need to be able to:

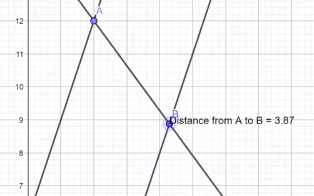
You can download the interactive used in the video (Geogebra file)

Answer to the textbook question at the top of this page